One of the most common criticisms of how Maths is traditionally taught at school is that it does not incentivise the search for creative and imaginative solutions enough. In fact, formal Mathematics is very often perceived as an enemy of artistic thought. Many associate the subject with boring, repetitive algorithms, and remember having to solve the same exercises over and over again in their dull little exercise books.
However, this divergence between Maths and Art is, in fact, artificial and has to do with the way we were taught. History has kept record of numerous artists who teased out the connections between the two disciplines, and there are many mathematicians who believe that beauty, inspiration, or creativity play key roles in their work.
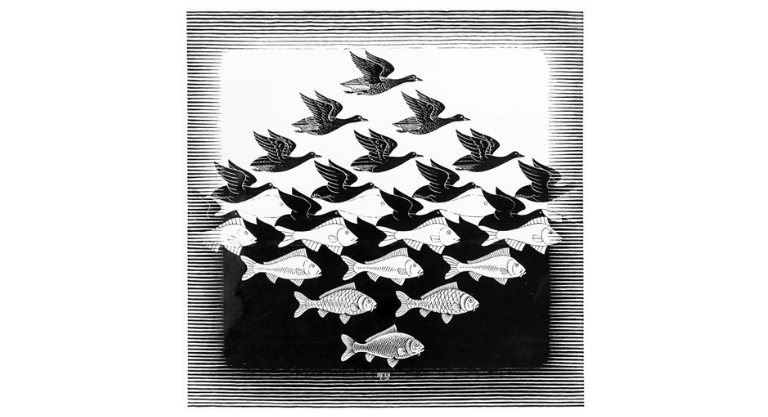
Maurits Cornelis Escher (1898-1972) is, probably, one of the most representative examples of this riveting relationship between Maths and Art. His work is a never-ending collection of impossible perspectives, ingenious games, endless tessellations and geometric provocations. This summer (2021), Drassanes de Barcelona hosted an exhibition that united the artist’s most representative works until the 26th of September. Laura Morera visited it with her sons and, aside from highly recommending it to us, kindly provided us with photos she took while there.

In Mathematics, we say that a shape tiles (or tessellates) the plane if, on inserting identical copies of itself—without overlapping nor leaving any gaps between them—, we are able to cover an endless plane. It is true that Escher took certain liberties in his tessellations, subtly changing each iteration in order to obtain a final shape that is quite different from the original one. Thanks to these transformations, he constructed the Metamorphoses, which include Sky and Water, the famous woodcut (Fig.1). Even though, strictly speaking, these works are not tessellations, their geometric construction is fascinating. Do you think that all polygons are able to tile the plane? What characteristics must they possess in order to do so? Let’s investigate!
This sample provides an excellent opportunity to stimulate the more creative students with more artistic sensitivity or, perhaps, those who feel less drawn to Maths for various reasons. From all of Escher’s works, the tessellations are a good choice for the classroom, thanks to their geometric simplicity as well as the visual impressiveness of the results.
In the case of younger children, it might be a good idea to begin with a very simple polygon: the triangle. If we ask the children to draw any triangle, we can encourage them to check if endless repetitions of the triangle tile the plane. This presents itself as a good opportunity to work on conjectures in the classroom: the children will discover that all the triangles they could draw tile the plane, but it is important to highlight that a few examples are not enough to affirm that it can be achieved with any triangle. Some GeoGebra applets, like this one, can help to visualise that any triangle can, indeed, tile the plane. However, these visualisations help us to show, not to prove. If we believe our students are ready, we can try to come up with a logical reason all together that allows us to justify why any triangle has the property of tiling the plane. In this way, we can get closer to a proof.

In any case, it can be very productive to extend the same exercise to more complex polygons, depending on the students’ age and abilities. Quadrilaterals can also tile the plane, even though the formal proof may be too tedious for the classroom. For polygons with more sides, the tessellations become more complicated. In fact, out of all regular polygons, the hexagon is the only one that can tile the plane (aside from quadrilaterals and triangles, as we have already seen).
The case of the pentagon is particularly interesting, because it has been the subject of recent research in the mathematical community. The first irregular pentagons that tile the plane were not discovered until 1918 by the mathematician Karl Reinhardt, who defined five types. For half a century, no more were found; until, in 1968, the mathematician Richard Kershner discovered three new ones and conjectured that there were none left. In 1977 Marjorie Rice, a Maths amateur, refuted Kershner’s conjecture, discovering four more pentagons with this property. Gradually, more were discovered, up to a total of 14, but no one knew for certain if there were more to be found or not.

The mystery remained unsolved until 2015, when Casey Mann, Jennifer McLoud and David Von Derau discovered a fifteenth pentagon that was able to tile the plane. What’s more, they proved, with the help of a computer, that there are no more than fifteen possible pentagons. This result is truly fascinating: out of the infinite number of pentagons that can be generated, only fifteen—an apparently arbitrary number—are able to tile the plane. And we are now certain that there are none left to be found!
The example of the pentagon that is able to tile the plane can help us to convey an image of Maths as a living discipline that is constantly evolving. Although Mann, McLoud and Von Derau’s proof is of a very high level, it could be interesting to involve the students in the mystery that they solved, using it to show how conjectures are proved in Maths.
And how about you? Can you think of any other activities inspired by Escher’s work that you’d like to bring to the classroom? What else do you use as inspiration for your lessons?
Soon, in a new blog entry, we’ll explain how you can imitate Escher’s process of construction in the classroom in order to obtain spectacular tessellations.